I was at Costco Red Deer and came across the Complete Canadian Curriculum book for grade 5 math. I turned to the page on Fractions, and it's that familiar explanation again. Just like most search results on Google, it defines a proper fraction as one where the numerator is smaller than the denominator. Is it incorrect? Well, not entirely wrong – but it falls short when it comes to helping kids truly get the hang of fractions. The definition is not helping kids understand why fractions work the way they do.

Worksheet from the Complete Canadian Curriculum.
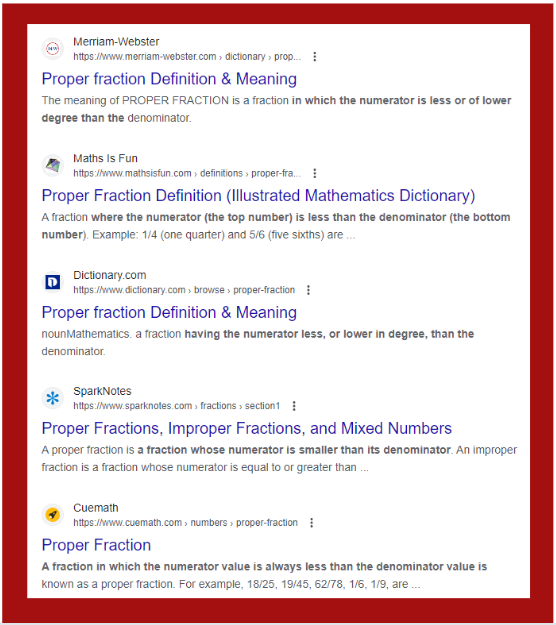
Results from Google search.
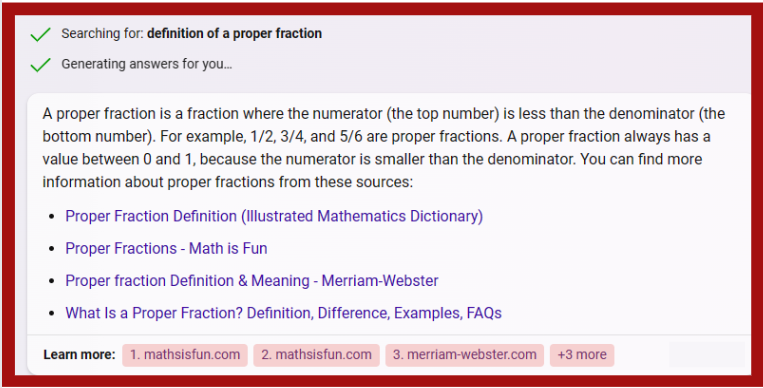
Another one from Microsoft Bing.
How Mathnasium Explains: conceptual, not procedural – and keep it simple!
At Mathnasium, we take a different approach: we're all about understanding the concepts, not just the steps. So, what's the right way to define a proper fraction? A proper fraction is a fraction whose value is greater than zero and less than one. And here's our simple addition to make it clear: "If you don't have all the parts, then you have a proper fraction" (check out the picture below). We're all about plain language that's easy to grasp, rather than using tricky terms like “numerator” and “denominator”, which even some grown-ups get mixed up. Let's dive into how Mathnasium breaks down the basic concept of fractions:
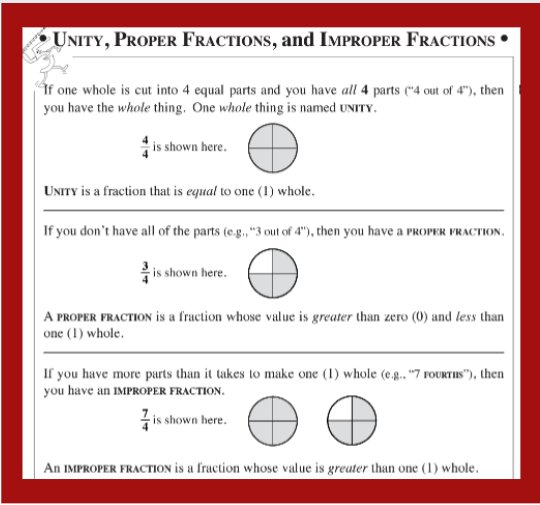
Zero and one – these are two of Mathnasium's key benchmark numbers (you might want to check out THE SEVEN BENCMARK NUMBERS – WHY IS THIS A POWERFUL TOOL FOR PRIMARY GRADES?. Getting a solid grip on what zero and one mean, and all the numbers that fall in between, lays a sturdy foundation for understanding fractions and decimals. This understanding sets the stage for tackling more advanced math challenges with ease. It's like building a strong base for even trickier problems down the road.
The idea that a proper fraction has a smaller numerator than the denominator could be more like a little extra insight, not the main definition itself. The real focus needs to be on understanding the true value of a fraction, rather than just following the steps. Now, you might wonder, "Why make a fuss? If it's not incorrect, it should do the job." Well, here's the kicker – while it might not bother us grown-ups, it matters a whole lot when it comes to teaching kids. Our teaching should match their way of thinking, not ours. Mathnasium's approach, rooted in making concepts crystal clear and putting them in plain language, has a bigger impact on how well students grasp numbers. It's all about building that strong sense of numbers from the ground up.

Exploring fractions, one slice at a time!
Conclusion – and it is not just about definition of a proper fraction
This is just one example of a math definition that many educators teach students. While the definition itself is not wrong, it falls short on capturing the complete essence and depth of the concept. In the world of education, don’t underestimate the power of precise definition in math. We acknowledge that guiding students and making them understand the underlying concept demands more time, but it is the pivotal moment that shapes a student’s lifetime approach to math: will they evolve into a creative, critical thinker and mathematical enthusiast, or will they merely be a ‘math machine’ adhering to steps and procedures?

















