The Beauty Of Maths Patterns: Exploring Mathematical Sequences
The Beauty of Maths Patterns: Exploring Sequences | Mathnasium
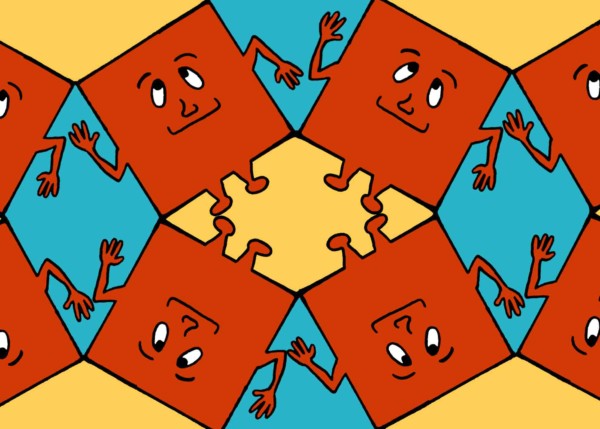
This month, we're celebrating math in all its beauty, and we couldn't think of a better topic to start than tessellations! A tessellation is a special type of tiling (a pattern of geometric shapes that fill a two-dimensional space with no gaps and no overlaps) that repeats forever in all directions. They can be composed of one or more shapes... anything goes as long as the pattern radiates in all directions with no gaps or overlaps. You can find tessellations of all kinds in everyday things—your bathroom tile, wallpaper, clothing, upholstery... and even in paper towels!
Because tessellations repeat forever in all directions, the pattern can't have unique points or lines that occur only once, or look different from all other points or lines. Additionally, a tessellation can't radiate outward from a unique point, nor can it extend outward from a special line.
While any polygon (a two-dimensional shape with any number of straight sides) can be part of a tessellation, not every polygon can tessellate by themselves! Furthermore, just because two individual polygons have the same number of sides does not mean they can both tessellate. In a tessellation, whenever two or more polygons meet at a point (or vertex), the internal angles must add up to 360°. Only three regular polygons (shapes with all sides and angles equal) can form a tessellation by themselves—triangles, squares, and hexagons.
What about circles? Circles are a type of oval—a convex, curved shape with no corners. Circles can only tile the plane if the inward curves balance the outward curves, filling in all the gaps. While they can't tessellate on their own, they can be part of a tessellation... but only if you view the triangular gaps between the circles as shapes.
There are three different types of tessellations (source):
Tessellations figure prominently throughout art and architecture from various time periods throughout history, from the intricate mosaics of Ancient Rome, to the contemporary designs of M.C. Escher. Get creative and try making tessellated masterpieces of your own using this handy tessellation creator (courtesy of the National Council of Teachers of Mathematics)!


Mathnasium meets your child where they are and helps them with the customised program they need, for any level of mathematics.
